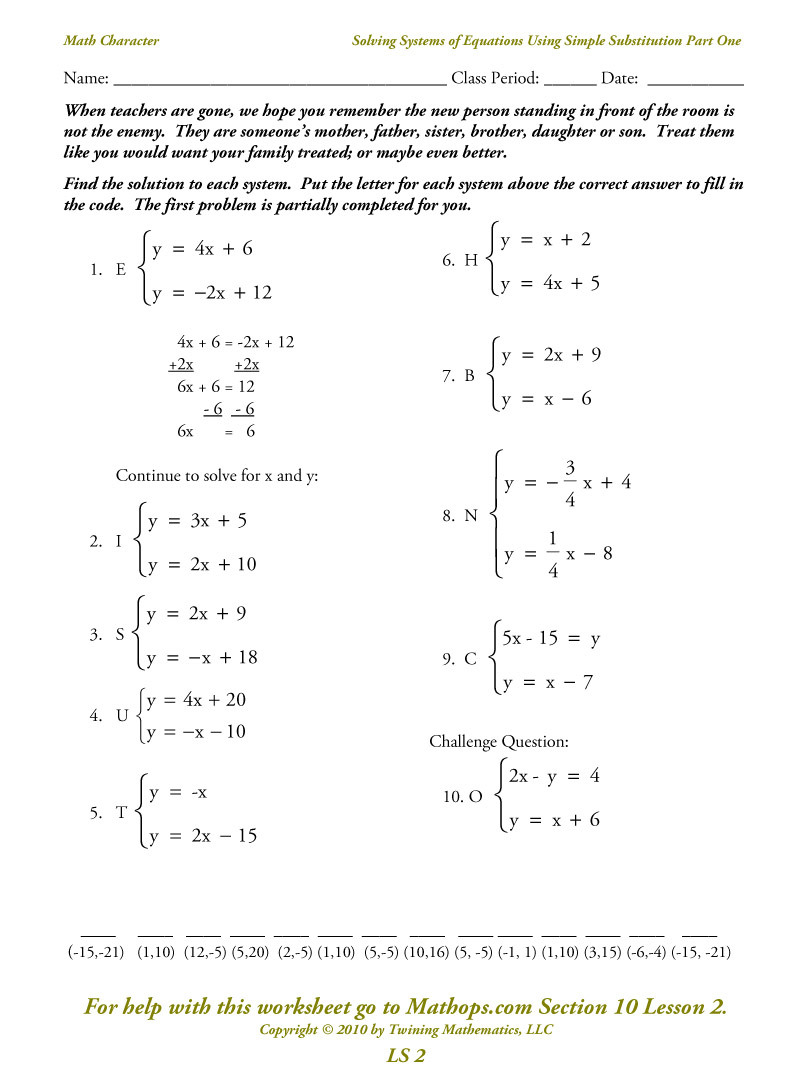
vortiru.blogg.se - Substitution equation systems

SUBSTITUTION EQUATION SYSTEMS HOW TO

To understand this well, let us solve some examples. When we are solving systems of linear equations by substitution, typically, one equation and one of the variables pave the way for the solution more quickly than the other. Such an equation must involve both variables. We can do so by putting them into either of the original equations. Step 4: Solve the second equation to find the value of the other variable. Step 3: Substitute the variable’s value (solved in Step 2) in the other equation. Step 2: Now, we have to solve it for any of its variables (say, either x or y). Step 1: Select one equation from a pair of linear equations. Then, we will do the substitution.įor solving systems of linear equations by substitution, we have to follow these steps: There is no value for either x or y available for substitution.

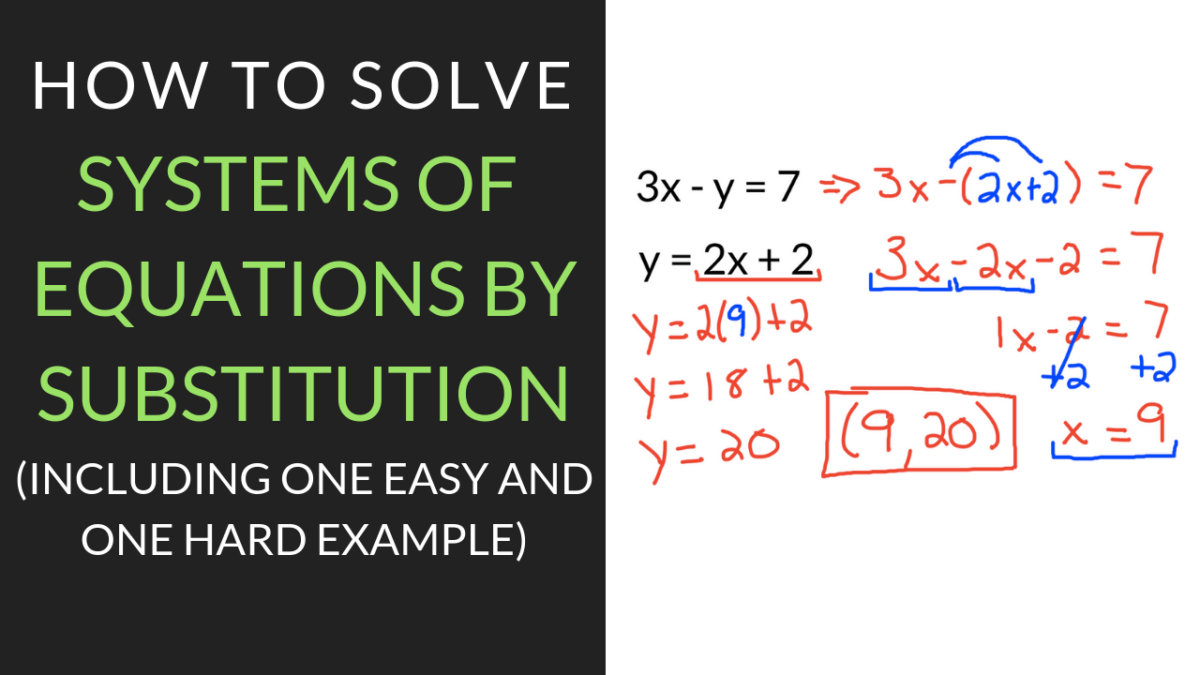
Here, we can observe that none of the above equations is already solved. Rather, you will get the problem as a pair of linear equations to solve. There will be no ready-to-use value for substitution. Usually, as a High school student, you won’t get the value of x or y already equated. Solving for a variable first before using the Substitution Method So you can easily understand the concept. The above system of equations is a basic example. For this, we have to put the solutions back into the system of equations. It is better if we check these solutions. The solution to the given system of equations is (4,8). So, in solving the system of equations by substitution, we got our solution. For this, we will put the value of x into the 1st equation. But don’t forget, you need the value of y. Substituting the value of y in 2nd equation, When an equation has one variable, we can easily find its solution. Putting such a value of y will make the entire equation involve one variable. So, let us put the value of y in the 2nd equation. This means we can put this value in place of y. Here, the 1st equation shows that y is equal to 2x.

SUBSTITUTION EQUATION SYSTEMS HOW TO

(iii) A true statement, i.e., infinite solutions How to Solve System of Equations by Substitution (ii) An untrue statement, i.e., no solutions

(i) Only one value for each variable within the system, i.e., one solution Solving a system of equations by substitution method will have one of these results: Such equations of a system are independent.

Sometimes, the equations in a system do not share all solutions.

(It means they both represent the same line.)

When all the solutions of one equation are the solutions of the other equation, the equations are dependent.

The system having no solution is an Inconsistent solution.

The system having at least one solution is a Consistent solution.

Such a solution makes both equations true. Generally, a solution of a system of equations in two variables is an ordered pair. Systems of equations come as math problems, including two or more equations. It means two equations represent a similar line.

The lines intersect at infinite points.

Given two variables (x & y), the graph of a system of two linear equations is a pair of lines in the plane. It is a set of two or more linear equations. Notably, solving systems of equations by substitution method is very uncomplicated.īefore discussing how to solve the system of equations by substitution, let us crisply review the system of equations. The Substitution Method is highly useful in topics including linear algebra, computer programming, and more. We substitute one variable with its found value to solve the problem. Substitution involves putting one equation into another as the substitute of a variable. It is the method of solving systems of linear equations by substitution. What is the Solving System of Equations by Substitution? We will discuss what the substitution method is and how to solve system of equations by substitution. In this article, we will review the method of substitution. It is most easily applicable to systems of linear equations. Solving System of Equations by Substitution method is useful for solving a system of equations.